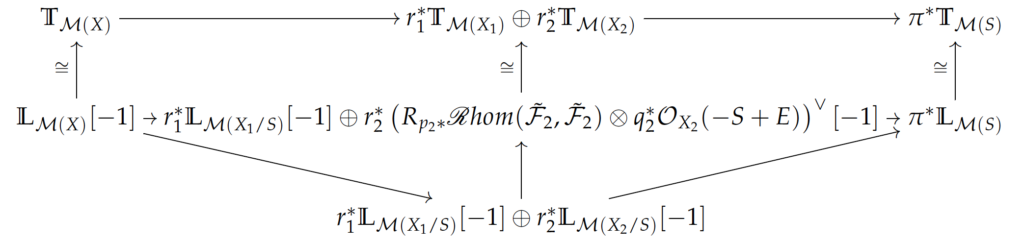
In the Spring 2019 Semester, the CMSA will be hosting a special lecture series on Derived algebraic/differential geometry run by Artan Sheshmani, with lectures given by Prof. Sheshmani and Dr. Dennis Borisov. The seminar will be held on Tuesdays and Thursdays from 3:00-4:30pm in CMSA, room G10.
Schedule:
Section 1: Basic setting of derived geometry
The goal: To collect the minimum set of tools needed to do algebraic geometry in the derived context.
| 2/05/2019 | Lecture 1: Model and с-categories | Video |
| 2/07/2019 | Lecture 2: Grothendieck topologies and homotopy descent | Video |
| 2/12/2019 | Lecture 3: Derived Artin stacks | Video |
| 2/14/2019 | Lecture 4: Cotangent complexes |
Section 2: Loop spaces and differential forms
The goal: This is the algebraic heart of the course – here we learn the homological techniques that are needed for shifted symplectic forms.
| 2/19/2019 | Lecture 5: De Rham complexes and S1-equivariant schemes (loop spaces) | Video |
| 2/21/2019 | Lecture 6: Chern character | Video |
| 2/26/2019
Room G02 |
Lecture 7: Local structure of closed differential forms in the derived sense Part I | Video |
| 2/28/2019 | Lecture 8: Local structure of closed differential forms in the derived sense Part II | Video |
| 3/05/2019 | Lecture 9: Cyclic homology | Video |
Section 3: Shifted symplectic structures
Goal: To see applications of the algebraic techniques from above in the geometric context of the actual moduli spaces.
| 3/07/2019 | Lecture 10: Definition and existence results | Video |
| 3/12/2019 | Lecture 11: Lagrangians and Lagrangian fibrations | Video |
| 3/14/2019
Room G02 |
Lecture 12: Lagrangians and Lagrangian fibrations | Video |
| 3/26/2019 | Lecture 13: Intersections of Lagrangians | Video |
| 3/28/2019
Room G02 |
Lecture 14: Examples and applications 2 (Part I) | Video |
| 4/02/2019 | Lecture 15: Examples and applications 2 (Part II) | Video |
Section 4: Uhlenbeck–Yau construction and correspondence
| 4/04/2019 | Lecture 16: Examples and applications 2 (Part III) | Video |
| 4/09/2019
Room G02 |
Lecture 17: Uhlenbeck–Yau construction and correspondence Examples (Part I) | Video |
